
This section briefly recalls the discretization scheme as described in more detail
in [18].
Some of the basic ideas of this discretization scheme have been formerly outlined by
Kraft [14] and
Hargraves and Paris [11].
A discretization of the time interval

is chosen.
The parameters 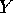 of the nonlinear program are the values of control and state variables
at the grid points
of the nonlinear program are the values of control and state variables
at the grid points 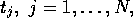 and the final time
and the final time 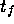
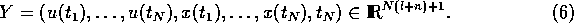
The controls are chosen as piecewise linear
interpolating functions between 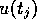 and
and  for
for 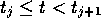
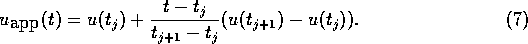
The states are chosen as continuously differentiable functions
and piecewise defined
as cubic
polynomials between
 and
and 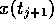 with
with 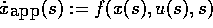 at
at 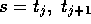 ,
,
The approximating functions of the states have to satisfy the differential equations (2)
at the grid points 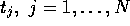 , and at the centers
, and at the centers 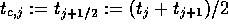 ,
, 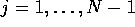 ,
of the discretization intervals.
This scheme is also known as cubic collocation at Lobatto points.
The chosen approximation (8) -- (12) of
,
of the discretization intervals.
This scheme is also known as cubic collocation at Lobatto points.
The chosen approximation (8) -- (12) of 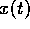 already fulfills these constraints at
already fulfills these constraints at  .
Therefore, the only remaining
constraints in the nonlinear programming problem are
.
Therefore, the only remaining
constraints in the nonlinear programming problem are
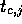
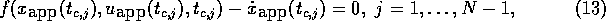
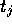
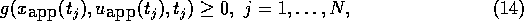
 and
and 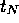

a reduced number of constraints to be fulfilled and
a reduced number of free parameters to be determined
by the numerical procedure.
This results in a better performance of an implementation of this method
in terms of convergence, reliability, and efficiency compared with other schemes.